From time to time, belltuners attempt to tune the upper partials in bells – those higher in frequency than the nominal. Most of the strong partials above the nominal are rim partials, i.e. they have an antinode or circle of maximum amplitude in the soundbow. The rim partials are strongly stimulated by the clapper, and together create the strike pitch of the bell. Investigations suggest that independent tuning of the rim partials is not possible. The chart of the rim partials for more than 8,000 bells of all ages and founders in my article on the upper partials of bells shows them all changing together in a very predictable way, and explains why this is so. Of course there are partials other than the rim partials above the nominal. These don’t contribute to the strike pitch but can affect the timbre of the bell if they are strong.
The motivation for attempts to tune the upper partials is that typically they do not form exact musical intervals with the nominal and the lower partials. For example, in the rim partials, if the superquint is an exact fifth above the nominal the octave nominal will be sharp of the octave. Over the years, much time has been spend and much bell metal removed in an attempt to bring all the upper partials into musical alignment in particular bells. A notable example is the Berlin Freedom bell, cast and tuned by Gillett and Johnston in 1951, where 1.4 tonnes of metal was removed from the 11.075 tonne bell without succeeding in bringing the upper partials into the desired relationship.
More recently, in 1971, similarly extensive tuning was done by John Slater of Whitechapel Bellfoundry on the tenor for Westminster Abbey, in an attempt to make this bell as perfect as possible. The tuning records for the bell give frequency measurements taken at the end of each of 19 cuts. The bell was tuned down from 36cwt 0qtr 23 lbs to 30cwt 1qtr 15lbs, i.e. 1829.3kg to 1543.6kg, removing 16% of the bell’s weight. The 19 sets of frequency figures mean that the effect of each tuning cut on many partials can be tracked. It is unusual to have such a detailed record of all the partial frequencies across such a large number of tuning steps, so the tuning records for this bell are of particular interest.
Throughout this paper, and in much of my work, the frequencies of partials are given as the interval in cents of the partial to the nominal partial. The nominal is the strongest determinant of the strike pitch (the note we hear) in bells. Representing partial frequency in this way means that the musical significance of the partial in the sound of the bell is readily apparent. Analysis of the partial frequencies in this way also demonstrates the strong relationships between the frequencies of the rim partials.
Tuning figures for Westminster Abbey tenor
I took recordings of the Westminster Abbey bells on 15 August 2019. Analysis of the sound of the tenor bell from these recordings gives tuning figures that correspond very closely to those taken in the foundry in 1971, but also allow us to see which partials are musically important, i.e. have a high intensity in the sound. The as-shipped tuning figures from the Whitechapel book and the figures measured from the recording are shown in the table below, together with comments on their amplitude in the recordings.
| Partial | Whitechapel figures | Recording 15/8/2019 | Comments | ||
|---|---|---|---|---|---|
| Freq. | Cents to nominal | Freq. | Cents to nominal | ||
| Hum | — | — | 147.1 | -2397.3 | Hum figures not available |
| Prime | 293.7 | -1200.0 | 294.1 | -1197.9 | |
| Tierce | 349.2 | -900.0 | 348.5 | -904.1 | |
| Quint | 440.0 | -500.0 | 440.6 | -498.1 | |
| Nominal | 587.3 | 0.0 | 587.5 | 0.0 | |
| 731.9 | 380.9 | 731.0 | 378.3 | Very weak | |
| 747.8 | 418.1 | 747.1 | 416.0 | Weak | |
| 757.0 | 439.2 | 757.7 | 440.4 | Stronger | |
| — | — | 823.7 | 585.0 | Weak | |
| Superquint | 880.0 | 700.0 | 879.7 | 698.9 | Rim partial |
| 960.6 | 851.7 | 959.9 | 849.2 | Weak | |
| 1060.2 | 1022.6 | — | — | Undetectable in recording | |
| — | — | 1108.5 | 1099.1 | Weak | |
| Octave Nominal | 1211.4 | 1253.3 | 1212.5 | 1254.3 | Rim partial |
| I7 | 1573.5 | 1706.0 | 1574.5 | 1706.5 | Rim partial |
| I8 | 1959.5 | 2085.9 | 1962.0 | 2087.5 | Rim partial |
| I9 | 2363.0 | 2410.1 | 2364.0 | 2410.2 | Rim partial |
| I10 | — | — | 2776.5 | 2688.7 | Rim partial |
Because of the good correspondence between the frequencies measured in the recording and those taken by Whitechapel, I have not made any compensation for recorder speed. A previous comparison with youtube videos with the sound of the bell being tolled full-circle for the funerals of Diana, Princess of Wales on 6 September 1997 and the Queen Mother on 9 April 2002 showed the same close correspondence after adjustment for recorder speed variation.
The figures show that the rim partials, although in a rough harmonic series so as to generate the strike pitch, do not form exact intervals with the nominal. This is always the case in bells of normal shape. The superquint is tuned exactly to a fifth above the nominal, and as a result the octave nominal is 54 cents or half a semitone sharp. Had the octave nominal been an exact octave above the nominal (which would have implied an abnormally thick, heavy bell for a change-ringing tenor) the superquint would have been flat. The I9 partial is tuned close to the double octave but this is the fifth partial above the nominal, whereas the harmonic partial at the double octave is the sixth above the nominal, not the fifth.
Sound spectrum of Westminster Abbey tenor
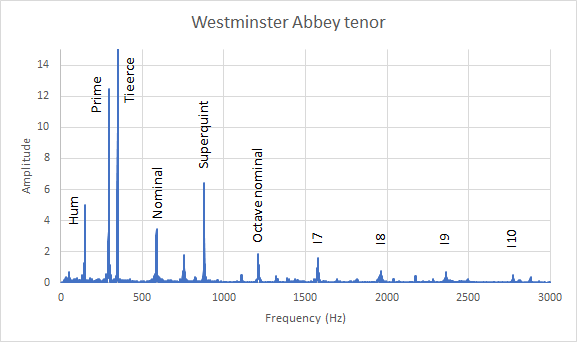
The first chart below is the spectrum of the bell sound showing all the measured partials.
The second chart is the spectrum for the frequencies between nominal and octave nominal where many of the partials recorded by the tuner lie.
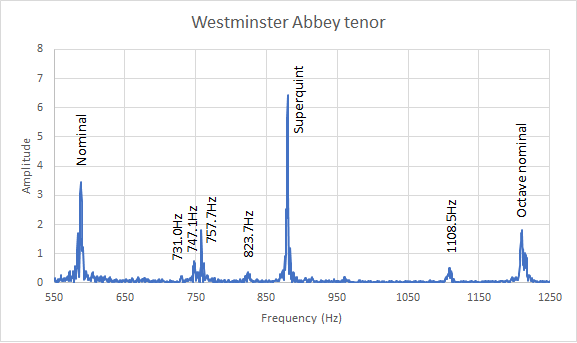
Points of interest in these spectra include:
- I could find no trace in the recording of the partial at 1060.2Hz noted by the tuner
- The weak partials at 823.7Hz and 1108.5Hz were not recorded by the tuner
- The partials at 731.0Hz, 757.7Hz and 959.5Hz are quiet and don’t significantly affect the sound of the bell.
Most bells from all founders have a group of three or four observable partials between nominal and superquint, as does this bell. It is sometimes suggested that these closely spaced partials are a triplet of a single partial – that a single partial is split into three by asymmetry in the bell. However this is not the case, tapping round the rims of other bells using an adaptation of Rayleigh’s method shows they have different numbers of nodal and antinodal lines and are different partials. This is confirmed by the experiments and partial classification schemes of Perrin, Charnley and De Pont, and of André Lehr.
Effect of tuning on partial frequencies – rim partials
As mentioned in the introduction, it is unusual to have a detailed record of all partial frequencies across all tuning steps. I have quite a few recordings or tuning figures of bells before and after tuning in my collection, but two other bells where special efforts were made by the tuner to tune the upper partials are the bass bell of the carillon at Culver of 1950, and the Berlin Freedom bell of 1951 mentioned in the introduction. Both are Gillett and Johnston bells and the before-and-after figures are available from the G&J tuning books. I have included the figures for these bells in many of the charts below.
The first partial to look at is the octave nominal, or rather the interval in cents between octave nominal and nominal, as the tuning cuts progress. This interval is a good measure of the relative thinkness and weight of a bell. What the chart below shows is a series of heavy cuts taking a lot of metal out of the bell on each cut up to cut number 5, followed by a series of lighter cuts during which the octave nominal continues to get sharper relative to the nominal up to cut 9. The rest of the tuning is a series of light cuts clearly attempting to tune other partials, giving minor variations in the octave nominal interval.
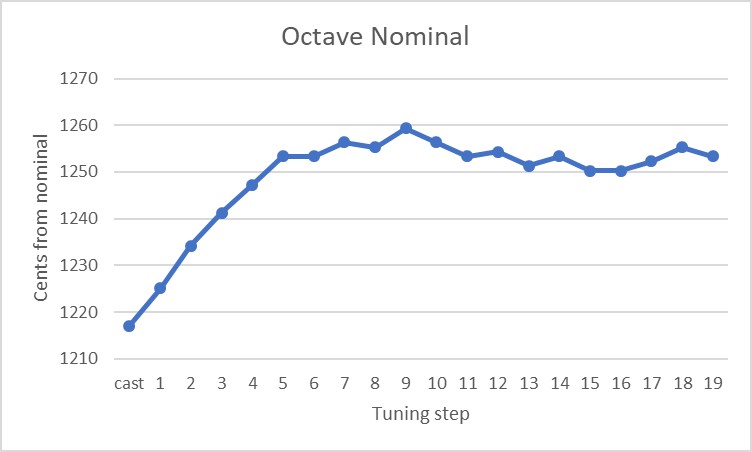
For the rest of the rim partials, what we expect to see is that the interval of each rim partial to the nominal moves in accordance with the interval of the octave nominal to the nominal, as predicted by the starbust diagram in the article on upper partials. Here are plots of the superquint, I7, I8 and I9 against the octave nominal. In the last few tuning cuts, the superquint landed exactly a fifth above the nominal. There is no need to include charts for nominal and octave nominal because these are straight lines due to the way the intervals are calculated. These charts also show the before and after figures for the Berlin Freedom bell and the bass bell of the Culver carillon, and a regression line taken from 6,100 bells.
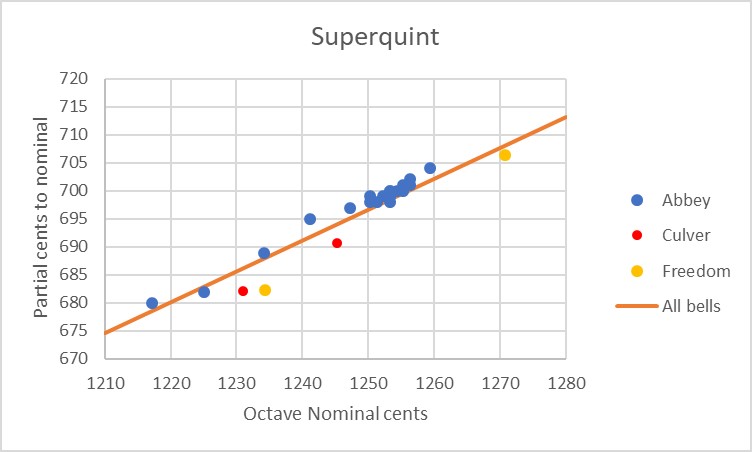


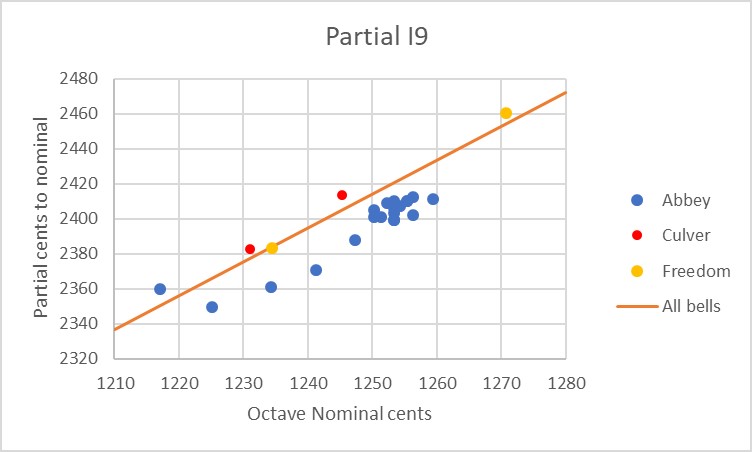
The linear relationship between the rim partials and the octave nominal in all the bells is clear. Despite the efforts of the tuner on this bell it wasn’t possible to independently change any of the higher rim partials – they all moved together.
The tierce behaves differently. The tierce has the same pattern of nodal and antinodal circles around the bell as the other rim partials, so in principle it would also move proportionately to the octave nominal. However, because it is the rim partial with the simplest pattern of nodal lines, and also due to the exact location of the antinodal circles, the tierce interval to the nominal can move a little independently of the other rim partials. This is why it is possible to have bells of normal shape with sharper or flatter tierces. The other point to note is that because it is the other side of the nominal, it moves the other way to the other partials as the octave nominal changes. As metal is taken out, the rim partials move further apart, and so the partials above the nominal get sharper whereas the tierce gets flatter. The chart below shows a general drift down of the tierce as the octave nominal goes up, but the relationship is loose.
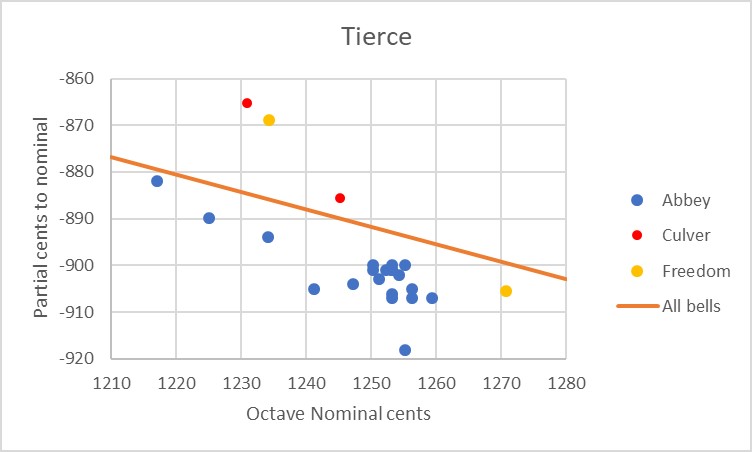
Despite the best efforts of the tuner, these plots show that it wasn’t possible to independently tune the rim partials above the nominal.
Effect of tuning on partial frequencies – prime and quint
The prime and quint have nodal and antinodal circles in different locations to the rim partials, and their relative frequencies are not dominated in the same way by the shape of the sound bow. Here is how they changed with the 19 tuning steps:
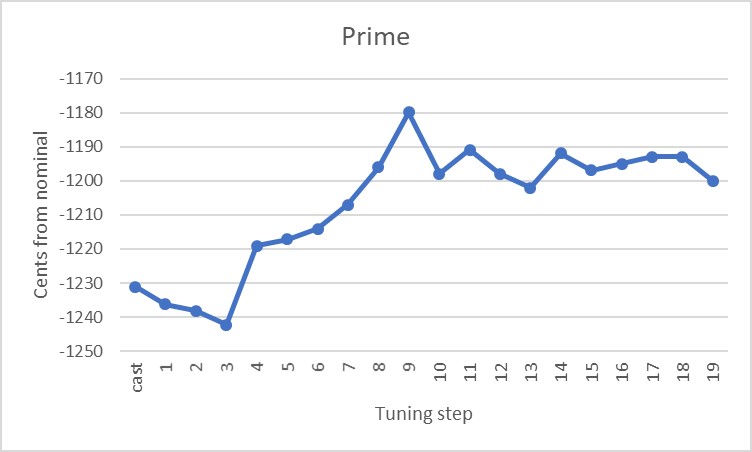
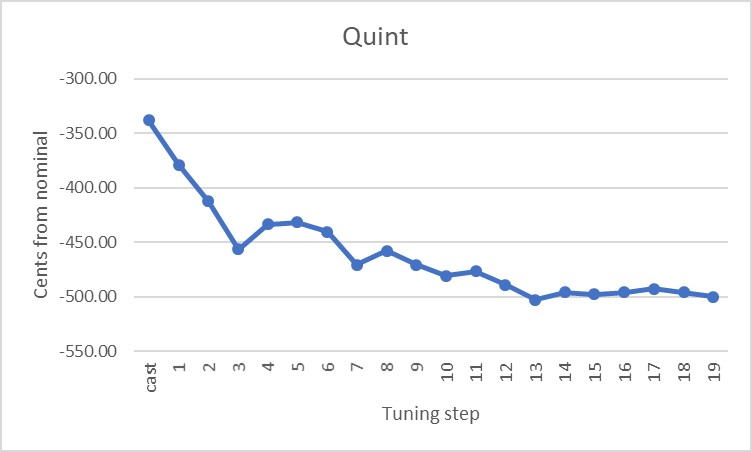
From tuning cut 9 or 10 onwards, both partials were close to the ideal intervals to the nominal of -1200 cents for the prime and -500 cents for the quint. With the final cut, the tuner landed both partials exactly on the ideal intervals.
Effect of tuning on partial frequencies – other partials
The loudest partial not yet considered is the strong partial at 747.8Hz, between the nominal and superquint, with an interval slightly sharp of a major third to the nominal. A partial as strong as this will affect the timbre of the bell.
The other partials not yet described are the partials at 731.9Hz, 757.0Hz, 960.6Hz and 1060.2Hz. All are low intensity and one is undetectable in the recordings. None make a significant contribution to the timbre of the bell because they are so weak. Here are charts showing how these partials change with each tuning step:
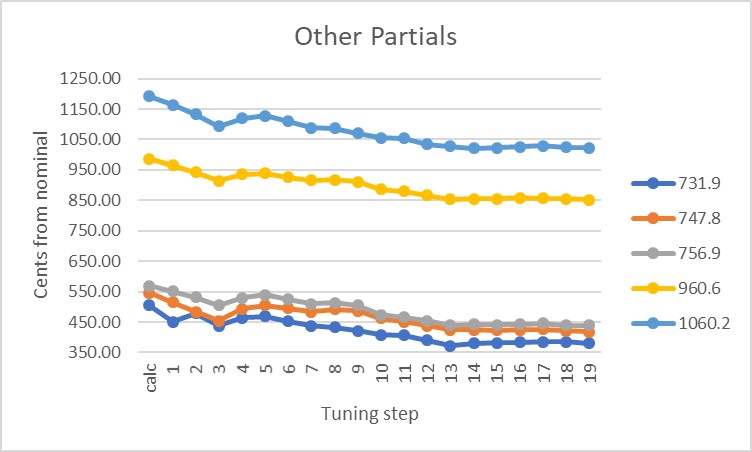
All five of these partials move together through the tuning process. The figures for the partial at 731.9Hz jump at tuning steps 1 and 2 but this may be due to measurement errors. Certainly from step 3 onwards all these partials move together. These partials in general have antinodal circles at different heights in the bell, so this is a slight surprise. What is common between all these partials which might explain why they tune together is that they all have a node (a circle of zero amplitude) at the soundbow. The correlation with the octave nominal is poor, due without doubt to the fact that their antinodal circles are at different heights in the bell to the rim partials.
Conclusions
It is unusual to have such detailed records of the tuning of a bell. John Slater set out to make this bell perfect – by implication, to tune the partials with frequencies above the nominal partial to exact intervals. Below the nominal, the tuning of prime and tierce are tuned exactly to the equal tempered intervals and the tuning of these partials was very successful. As regards the higher partials the picture is different. As metal was taken out of the bell, the rim partials (nominal, superquint, octave nominal, I7, I8 and higher partials) changed according to the expected relationship and the tuner wasn’t able to affect them independently. The superquint is tuned to an exact fifth above the nominal and as a result the octave nominal is half a semitone sharp. This can be predicted from the normal relationships between these partials.
Of the other partials whose frequencies were measured by the tuner during the tuning process, only one is musically significant and two can’t be detected at all in recordings of the bell. In any case, these partials were not tuned indepedently, they all moved together throughout the tuning process.