Around 20 years ago, I rang on the peal of eight at St Edmund Southwold. All the bells had been tuned in 1990 by Whitechapel with accurate nominals, but to my ears the trebles sounded distinctly flat, by up to a quarter of a semitone. The two trebles at Southwold cast by Moore, Holmes & Mackenzie in 1881 are thick and heavy, and the remaining bells are thinner, so that the treble is heavier than the third. On another occasion, I was walking past St Mary Magdalen, Oxford listening to the 2000/2001 trebles of the Taylor peal of ten. Again, these trebles sounded flat though their nominals are correct. Yet another example is the 2012 Whitechapel peal of twelve at High Wycombe. My investigation into why these bells and other thick and heavy trebles can sound flat despite accurate tuning led to my PhD research. This article updates the work based on a very much greater number of bell recordings.
Sometimes, peals of bells are tuned with stretch, so that the nominals of the trebles are sharper than the musical scale would require by a quarter or a third of a semitone. Stretch tuning was common in peals of bells produced before the true-harmonic revolution in the late 1890s. Paul Taylor re-introduced the practice at the Taylor bellfoundry from the early 1950s and it continued until the late 1980s (information from Mike Milsom). Some people like the effect, some don’t. Paul Taylor had a keen musical ear and would not have produced bells that to him sounded out of tune. But until I did my research there was not a good explanation of why he thought stretch tuning was desirable.
Virtual pitch theory, explained below, provides a good explanation of the strike pitch of bells (the note we hear when a bell rings) and why the frequency corresponding to the pitch that we hear need not be present in the bell sound. But theories can only be validated by their predictions. In this article, I use virtual pitch theory and the results of my PhD experiments to predict the stretch nominal tuning required for the strike pitches to sound in tune, for a number of Taylor peals from the 50s, 60s and 70s. There is good agreement between theory and practice which confirms the virtual pitch theory as an explanation of strike pitch.
At the end of this paper, I explain why stretch tuning of trebles involves a compromise between the flattening of the strike pitches of smaller bells due to their profile, and the sharpened partials in the small bells sounding out of tune with the larger bells.
Virtual pitch and the strike pitches of bells
Virtual pitch theory says that if a sound contains frequencies 2f, 3f, 4f, 5f etc. then a strong sensation of pitch corresponding to frequency f is generated in the ear or auditory system even if this frequency isn’t present in the sound. Experiments show that in bells the lowest frequency in the set – the frequency 2f – needs to be in a range of around 500Hz to 1500Hz for the strike pitch to be formed. The virtual pitch theory provides a robust explanation of the strike pitch in bells – the note that we hear when a bell rings, and also other effects such as secondary strike.
In a bell of normal western shape, there are a set of partial frequencies which always approximate to the frequencies required for virtual pitch or strike pitch generation. This set of partials, called the rim partials, have an antinode (a circle of maximum amplitude) in the soundbow of the bell, and one nodal circle (a circle of minimum amplitude) part-way up the waist. As their frequency increases, the rim partials have an increasing number of nodal and antinodal lines from rim to crown. Following the notation of André Lehr explained in Lehr’s paper on Dutch Bell-Founding and tuning, the rim partials with their conventional names are as follows:
| Name | Lehr notation | Nodal lines |
|---|---|---|
| Tierce | I3 | 6 |
| Nominal | I4 | 8 |
| Superquint | I5 | 10 |
| Octave Nominal | I6 | 12 |
| I7 | 14 | |
| I8 | 16 | |
| I9 | 18 | |
| I10 | 20 | |
| I11 | 22 |
Here is a picture of the tierce, with a nodal circle in the waist of the bell and six nodes and antinodes around the rim:

The rim partials are always prominent in the sound of a bell, and become more prominent when the bell is struck harder. This is because the antinode in the soundbow means that they are strongly stimulated when the bell is struck.
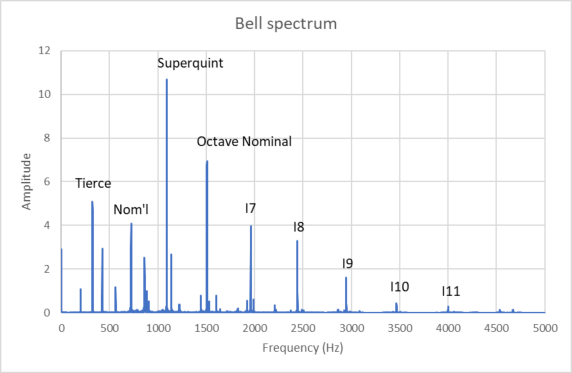
The frequencies of the partial series Nominal, Superquint, Octave Nominal, I7, I8 etc. are in the approximate ratios 2 : 3 : 4 : 5 etc. and create a virtual pitch of about half the nominal frequency, i.e. an octave beneath the nominal, which is the strike pitch we hear. In big bells, where the nominal is below 500Hz the ear can create a secondary strike pitch at a higher frequency based on higher frequency rim partials. The virtual pitch generation mechanism in bells is robust against changes in amplitude and frequency of the partials that generate the strike pitch. In particular, the frequencies can differ from the ideal ratios by quite an amount, as shown in detail below.
Frequencies of rim partials
One of the areas I investigated in my PhD research was the effect of changes in rim partial frequency on the strike pitch of bells. If the frequencies of the rim partials could be varied independently this would require a large number of tests to establish the effects. However, in preparing this experiment I discovered the remarkable fact that all the frequencies of the rim partials are strongly related. When the frequency of one is changed, the changes in the frequencies of all the rim partials can be predicted. I am not aware that this was known before I discovered it.
A good way to represent the relationship is to consider the interval in cents between each rim partial and the nominal (chosen because it is the primary determinant of strike pitch). Theoretical investigations suggest that the logarithmic relationship implied by conversion of the intervals to cents is justified by the maths and physics of the vibrations, as explained in a paper I published with Bob Perrin.
The diagram below shows how the frequency intervals are related. The horizontal axis is the interval in cents between octave nominal and nominal. The vertical axis is the interval in cents between each partial and the nominal. As well as the rim partials, the other low partials (hum, prime and quint) are included. The hum, prime and quint are in different groups to the rim partials, i.e. they have different numbers and locations of nodal circles, so their frequencies are determined by different areas of the shape of the bell than the rim partials.
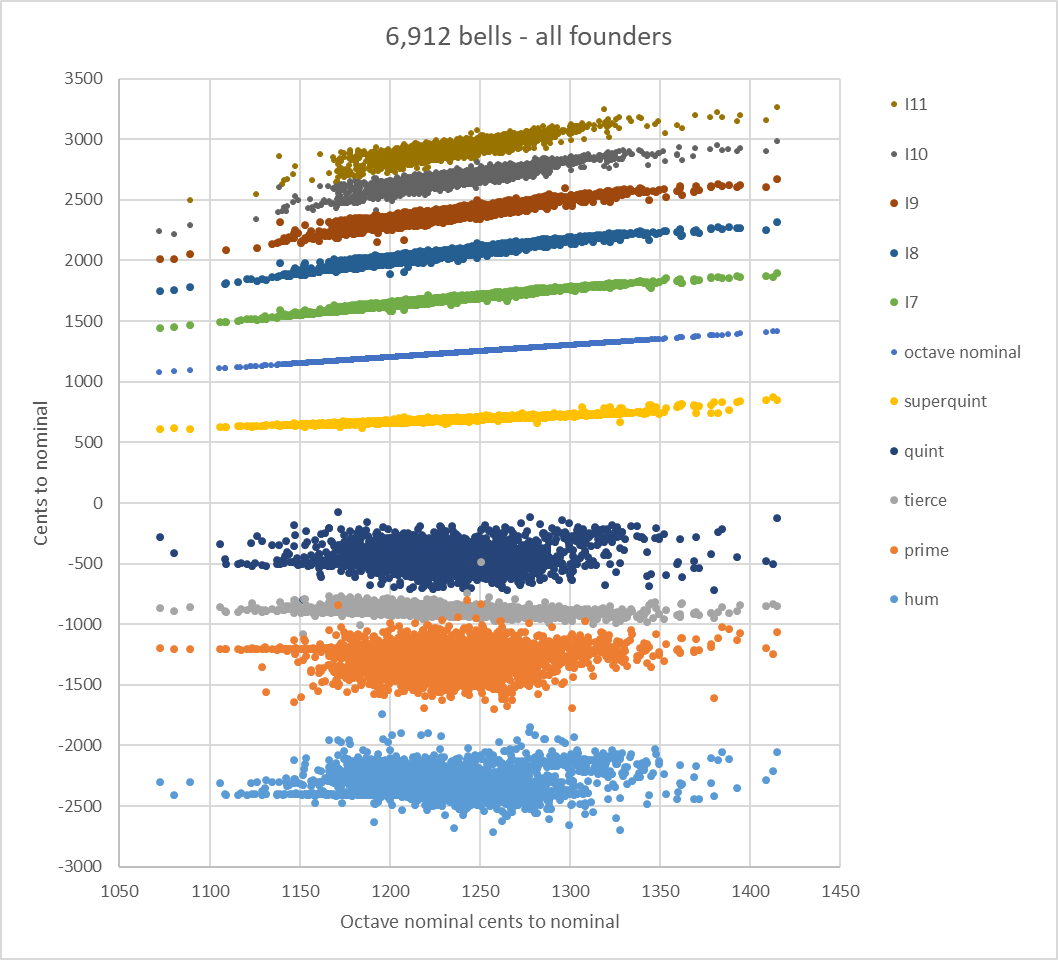
It is clear from this plot that there is a linear relationship between the interval cents of all the rim partials from nominal upwards. This plot comprises the tuning figures of 6,912 bells with dates from 1258 to 2018, weights from 1.8kg to 71.8 tonnes, from 29 different countries and 570 different founders, and includes 269 steel bells. Bell sound quality of these bells ranges from excellent to very poor. The chart shows that the relationship between the rim partial frequencies is always the same for bells of western shape.
The position of each bell along the horizontal axis is determined by the relative weight and thickness of the bell. If a bell is heavy or thick compared with other bells with the same nominal frequency, the intervals between the rim partials are smaller, and the bell lies towards the left hand side of the chart. If a bell is light or thin compared with others with the same nominal, it lies towards the right of the chart.
As explained above, the hum, prime and quint are in different groups to the rim partials, i.e. they have nodal and antinodal circles at different heights in the bell. Therefore their frequencies are not related to the frequencies of the rim partials and the plot shows a poor correlation between the frequencies of hum, prime and quint and those of the upper partials. What can be seen in the plot is straight lines at -500 cents for the quint, -1200 cents for the prime and -2300 to -2400 cents for the hum, where the bell has been shaped and tuned to give an old-style or true harmonic result.
Effect of tuning on upper rim partials
When a bell is tuned, metal is removed and the bell gets lighter and thinner and moves towards the right hand side of the chart. This consistent change in the rim partials before and after tuning is true for all bells for which I have investigated recordings or tuning figures before and after tuning. It should be clear that is it not possible for a bell tuner to independently tune the rim partials, despite many attempts to do this. An example of a failed attempt is the tuning of the Berlin Freedom Bell described in detail in my PhD thesis, based on tuning figures from the Gillett & Johnston books. Despite over 1.4 tonnes of metal being removed from the as-cast weight of 11.075 tonnes in an effort to tune the upper partials independently, the before and after figures sit on the straight lines.
The physical reason for this is not hard to understand. All the rim partials have the same pattern of nodal and antinodal circles, so removing metal from an annular ring inside or outside the bell will affect all the rim partials together. The exception to this in practice is the tierce. Although it too has the same nodal and antinodal circles, it is possible by changing the exact shape of the bell to affect its frequency a little without changing the other rim partial frequencies. Once the bell has been cast, the relationship between the tierce and the other rim partials is little affected by tuning. This can be seen in the chart. The general shape of the plot for the tierce is an upward slope to the left as all the rim partials move closer together – thicker bells have sharper tierces relative to the nominal – but there is also a horizontal line of points around 880 to 900 cents below the nominal, where the bell moulder has shaped the soundbow to give a just or equal tierce independent of the relative thickness of the bell.
Rim partials of Taylor bells
In the plot of 6,110 bells, the width of the lines gets broader for the higher frequency partials. This is due to the huge variation in profile and shape for these bells. The plot below shows the same analysis for 1,801 Taylor bells, dating from 1803 to 2019. Exactly the same effects are visible as for the bells from all founders. However, despite the bells being from three different foundry locations over 215 years the spread in the lines for the upper partials is rather less. The rim partial frequencies of Taylor bells are related in the same way as bells from all founders.

Variation of intervals in upper partials
It was mentioned above that creation of the strike pitch is robust against variation in the intervals between the rim partials. In very thick / heavy bells, such as the trebles at the Bullring and Winchester Cathedral, the interval from nominal to octave nominal can be as low as 1080 cents. For bells such as the 4th at Cambridge St Edward (which has been over-tuned), the second at Little Somerford and almost all Naylor-Vickers steel bells (which were cast very thin) this interval can be as high as 1350 cents. Here are the average intervals and frequency ratios based on nominal = 2.0 for three examples:
| Thick bell 1080 cents octave nom | Typical bell 1220 cents octave nom | Thin bell 1350 cents octave nom | ||||
|---|---|---|---|---|---|---|
| Partial | Cents to nominal | Ratio | Cents to nominal | Ratio | Cents to nominal | Ratio |
| Nominal | 0.0 | 2.00 | 0.0 | 2.00 | 0.0 | 2.00 |
| Superquint | 602.7 | 2.83 | 680.2 | 2.96 | 752.2 | 3.09 |
| Octave Nominal | 1080.0 | 3.73 | 1220.0 | 4.05 | 1350.0 | 4.36 |
| I7 | 1473.0 | 4.68 | 1663.7 | 5.23 | 1840.8 | 5.79 |
| I8 | 1800.3 | 5.66 | 2036.3 | 6.48 | 2255.4 | 7.36 |
| I9 | 2084.0 | 6.67 | 2355.8 | 7.80 | 2608.2 | 9.02 |
| I10 | 2336.7 | 7.71 | 2635.0 | 9.16 | 2912.0 | 10.75 |
| I11 | 2568.8 | 8.82 | 2882.4 | 10.57 | 3173.5 | 12.51 |
For very thick bells, the strike pitch is generated without ambiguity, but the closed-up upper partials makes the bells sound tonally quite different from the rest of the peal. For very thin bells, the mechanism in the ear that generates the strike pitch starts to break down. Such thin bells often have two strike pitches close together, a virtual pitch generated by the rim partials, and one based on hearing the prime, giving a characteristic twangy sound.
It is by no means necessary for all the rim partials to be present with significant amplitude for a virtual pitch to be generated. Other experiments show that the nominal, superquint and octave nominal alone are sufficent, and if more rim partials are present, partial amplitude of the superquint and octave nominal doesn’t make much difference.
Effect of upper partial frequency on strike pitch
As the strike pitch of a bell is generated in the ear of the listener, to find out how strike pitch is affected by the frequencies of the upper partials, it is necessary to experiment on people’s hearing – it is not possible to measure strike pitch independent of the listener. For example, it is not possible to hear beats between the strike pitch and a tuning fork because the strike pitch doesn’t exist as a physical frequency. The discovery explained in the previous sections means that an investigation of how the strike pitch of bells changes with upper partial frequency becomes much simpler. It is not necessary to vary the upper partials independently, they can all change together in the experiments.
In the work described in detail in my thesis chapters 7, 8 and 9 I used a set of artificially generated bell-like sounds with different nominal frequencies and rim partial spacing to investigate the effect of these variations on strike pitch. Experimental subjects were asked to compare different sounds and decided which were closest in pitch. The sounds were short (250ms) to encourage listening under similar circumstances to bells rung in changes. These short sounds encourage perception of virtual pitch rather than listening to individual partial frequencies. The simulated sounds included hum, prime and tierce and the experiments also investigated whether changes in these partials could affect the pitch heard.
30 people completed these experiments and the results showed beyond all reasonable doubt that upper partial spacing affected the pitch experienced by the listeners. The closer together the upper partials, the flatter the pitch experienced, and the wider apart, the sharper. The size of the effect changed with nominal frequency. It was also established that changing the lower partials also had a slight effect on the pitch experienced, but much less than the effect of the rim partials.
The graph below shows how much the virtual pitch, i.e. the strike pitch, changes in cents for each cent change in the octave nominal interval. The interval between nominal and octave nominal was used as a proxy for the spacing of all the upper partials as they all change together. The vertical axis displays how much the strike pitch moves away from the half-nominal for a one-cent change in the octave nominal to nominal interval, at a range of different nominal frequencies.

The pitch shift effect is much greater at lower nominal frequencies and this is to be expected. At low nominal frequencies, the ear responds much more to the upper partials. At high nominal frequencies, the ear responds more to the prime or hum in forming the perceived pitch. The size of the shifts is relatively large. At a nominal frequency of 1000Hz, a change in the interval between nominal and octave nominal of 100 cents will change the strike pitch by 25 cents – a quarter of a semitone. This is of the same magnitude as the effect I first heard at Southwold many years ago. A difference of 100 cents in the octave nominal interval, for example from 1280 cents in the tenors to 1180 cents in the trebles, is not at all unusual.
The dotted line on the chart is a regression line fitted to the experimental values. From this regression line (and similar lines with a much smaller effect for tierce, prime and hum) it is possible to calculate the pitch shift in any bell from its partial frequencies. In practice what is of interest is the difference in shift between trebles and tenors in a peal. That is what will make the trebles sound out of tune.
Comparison with results from other researchers
Other researchers have also attempted to measure the shift in strike pitch due to changes in various partial frequencies. Mike Greenhough, a now retired physics lecturer at the University of Cardiff, in 1976 measured the partial frequencies of two bells in a local church, and demonstrated by simulating sounds that the pitch of the bells depended on the spacing of the upper partials. Eggen and Houtsma did a more detailed set of experiments based on a digital recording of a Hemony bell, and manipulated the frequencies of one or more partials to create a large set of different bell sounds. Four test subjects then measured the pitch of each sound by adjusting a sine tone until it matched the pitch they heard.
In both cases, the bell sounds used to the test differed from the sounds which bells produce in practice. Mike Greenhough used partials that were equal in amplitude, and only included partials up to the octave nominal. Eggen and Houtsma used an actual bell sound with a nominal frequency of 1004.8Hz. The changes they made in partial frequencies did not take into account the relationship between the frequencies of the rim partials, probably because they were not aware of it. Because of this, their experimental results are not strictly comparable with the results presented above. However, estimating the experimental values from the graphs in their paper, the pitch shifts in cents they measure for a one-cent change in the interval between nominal and octave nominal vary between 0.04 and 0.55 with an average of 0.36 cents. The equivalent shift from my experiments for a bell with nominal 1000Hz is 0.25 cents.
Stretch tuning of Taylor peals
In ringing peals, especially for higher numbers of bells, the trebles are generally cast thick and heavy compared with the tenors. This is for mechanical reasons, to make the rotational dynamics of the bells more comparable across the peal, and also supposedly to make them more powerful. Small bells in carillons do not have such a big disparity in profile compared with the bass bells because the mechanical consideration is not relevant. The extra thickness and weight in the trebles of ringing peals causes flattening of the strike pitch. This can be compensated for by sharpening the nominal frequencies and all the partials in the bell.
As explained in the introduction, in the early 1950s Paul Taylor introduced the practice of stretch tuning of trebles to Taylor’s bellfoundry. Their first complete stretched peal is said to be Bengeo in Hertfordshire cast in 1951. The 12 bells at Evesham, cast earlier in the same year, have some stretch in the trebles, and I understand from Chris Povey that Paul Taylor offered to retune the trebles down to temperament if the result wasn’t satisfactory but this was not done. Mike Milsom tells me that Paul experimented with the retuning of old peals of bells in 1951, before and after Bengeo were cast and tuned, and finally settled on the degree of stretch to be used when St Nicholas, Pierhead, Liverpool were cast in 1952.
Recordings in my library of Taylor bells cast and tuned as complete peals of 8, 10 or 12 from the 1950s to the 1970s are as follows:
| Peal | Date | Number of bells |
|---|---|---|
| Cambridge St Andrew | 1956 | 8 |
| Tewkesbury | 1962 | 12 plus flat 6th |
| East Retford | 1968 | 10 |
| Houston, Texas, St Thomas | 1971 | 8 |
| Chester Cathedral | 1973 | 12 plus flat 6th |
All these peals are tuned in equal temperament.
The charts below were produced by calculating the pitch shift for each bell in the peal individually from its partial frequencies, normalising the results by allocating a shift of zero cents to the tenor (so all the shift appears in the trebles), and plotting the result against the actual stretch in the nominal of each bell from equal temperament.
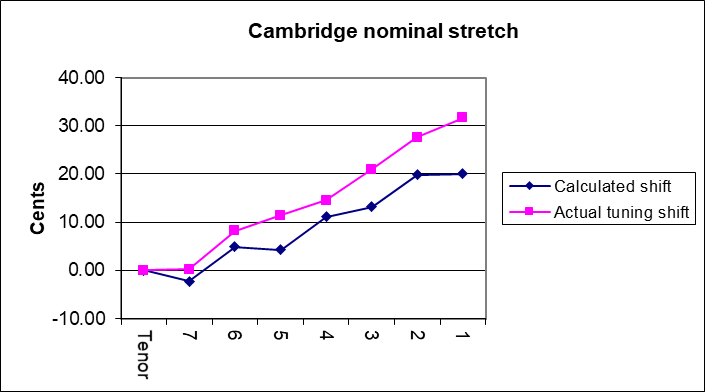
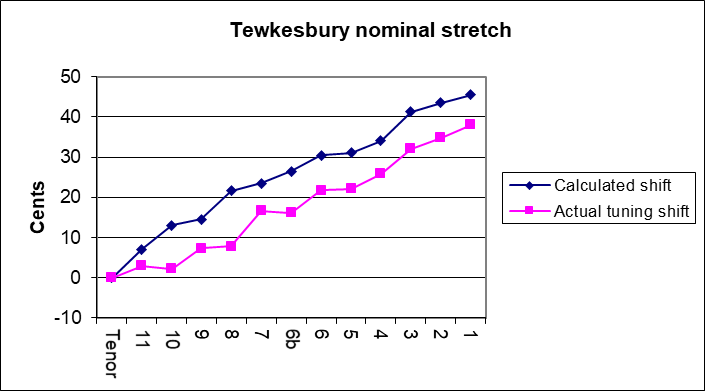
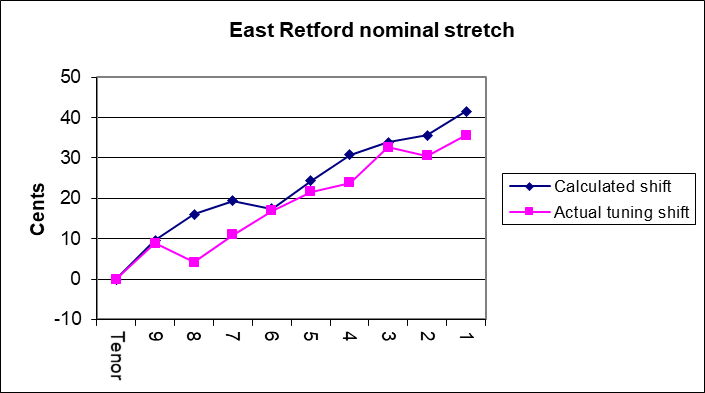
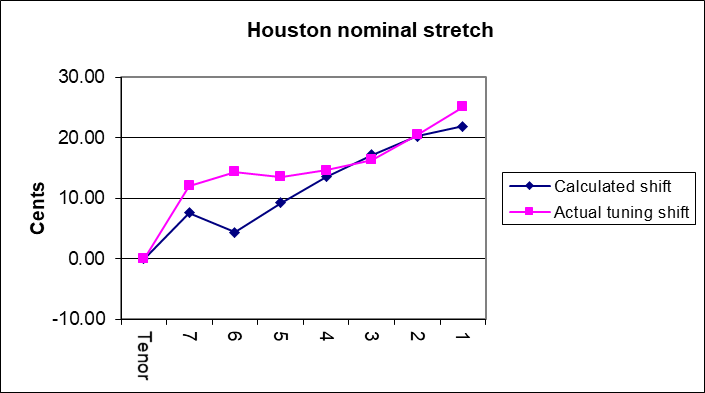
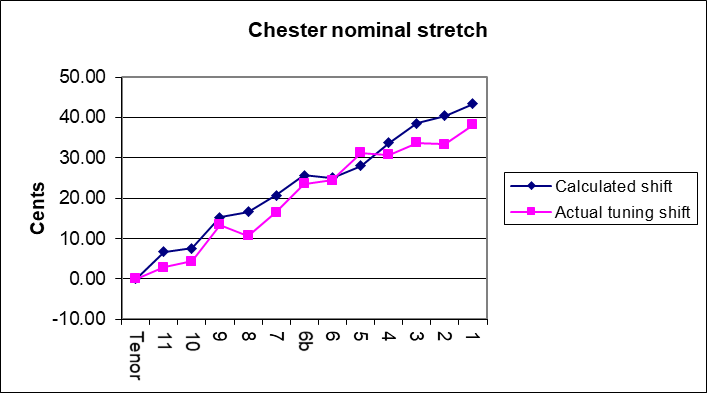
What is demonstrated by the analysis and these charts is a close agreement between the pitch shifts predicted by virtual pitch theory due to the bell design and partial frequencies, and the actual stretch in the bells as designed and tuned by Taylors.
This validates the virtual pitch theory as an explanation of the strike pitch in bells, and also gives a fascinating insight into what Paul Taylor heard when he listened to a bell.
Virtual pitch, spectral pitch and stretch tuning of bells
When bells are heard, there are two different modes of listening that occur depending on the circumstances and the listener. If a bell is listened to over a long period of time (seconds) and the listener has the skill, the individual partials are heard. The listener hears spectral pitch, i.e. the frequency of an individual partial. If the time for listening is short (for example if the bell is rung in changes) the ear tends to hear virtual pitch and assign a strike pitch based on the holistic effect in the listener’s ear. Bell tuners tend to hear spectral pitch, because that is how they tune bells.
When the profile of the trebles in a peal is different than the tenors, so that the trebles are proportionately thicker, then as shown in the last section the virtual pitch shifts flatten the strike pitch. If the nominals are tuned exactly to temperament the small bells will sound flat. This can be compensated for by sharpening the trebles. However, this means that someone hearing spectral pitch will say the front bells are sharp. The nominals, primes, hums and tierces of the smaller bells will be out of tune and sharp against the larger bells.
As a result of these two competing effects, a compromise is needed. The trebles need to be tuned sufficiently sharp that the virtual pitches don’t sound too flat, but not so sharp that the spectral pitches don’t sound too out of tune. The compromise is probably to split the difference slightly in favour of the spectral pitches, i.e. to tune the trebles sharp by a little less than half the virtual pitch shift. Some recent new peals of twelve that have been tuned on this principle include Great St Mary Cambridge, St Michael Cornhill and St Magnus Lower Thames Street.
Paul Taylor and stretch tuning
It seems obvious to me that Paul Taylor could hear virtual pitch accurately and used this skill to decide on stretch tuning for his peals. I don’t know how he measured the virtual pitches of his bells – it can only have been by some comparison method, as there is no way to measure virtual pitch directly with instruments. However he did it, his stretch tuning is vindicated by the results of virtual pitch experiments, and it is satisfactory to have an explanation, nearly 70 years later, of why it was employed.
Bill Hibbert
Great Bookham, Surrey
References and links
PhD Thesis, The Quantification of Strike Pitch and Pitch Shifts in Church Bells, W. A. Hibbert, April 2008
Partial Frequencies and Chladni’s Law in Church Bells, William A. Hibbert, David B. Sharp, Shahram Taherzadeh, Robert Perrin, Open Journal of Acoustics Vol.4 No.2(2014), Article ID:46533,8 pages DOI:10.4236/oja.2014.42007
Contemporary Dutch Bell-Founding Art, A Lehr, translated by R. Selman and T. Marton from “Hedendaagse Nederlandse Klokkengietkunst,” in Neth. Acoust. Soc. Publ. No.7, 1965, pp. 20-49
The Analysis and Synthesis of Bell Sounds, M. Greenhough, Proceedings of the Institute of Acoustics, Sept 1976, pp3-4-1 to 3-4-4.
The Pitch Perception of Bell Sounds, J. H. Eggen J. H. and A. J. M. Houtsma, Institut voor Perceptie Onderzoek, Annual Progress Report 21 (1986), pp15-23.
Bells and Bellfounding: A History, Church Bells, Carillons, John Taylor & Co, Bellfounders, Loughborough, England, Michael J. Milsom 2017, ISBN 978-1547239153
Master of my art: The Taylor bellfoundries 1784-1987, Trevor Jennings, John Taylor and Company (Bellfounders) Ltd, 1987, ISBN 978-0951198810